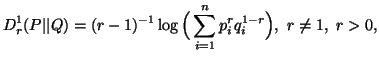
Rényi (1961) [82] first presented
a scalar parametric generalization of Kullback-Leibler's relative information
as
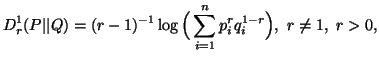
Rathie and Kannappan (1972) [79] presented an alternative way to generalize Kullback-Leibler's information as
![$\displaystyle D^s_s(P\vert\vert Q)=(1-2^{1-s})^{-1}\Big[\sum_{i=1}^n{p^s_iq^{1-s}_i}-1\Big],\s\neq 1,\ s>0,$](img936.gif)
Sharma and Mittal (1977) [91] studied
one and two scalar parametric generalizations of ![]() as
as
![$\displaystyle D^s_r(P\vert\vert Q)=(1-2^{1-s})^{-1}\Big[\Big(\sum_{i=1}^n{p^r_iq^{1-r}_i}\Big)^{s-1\overr-1}-1\Big],\ r\neq 1,\ s\neq 1,\ r>0,$](img939.gif)
Instead studying the measures ![]() ,
,![]() ,
, ![]() ,
, ![]() and
and ![]() separately, one can study them jointly. Let us write these measures in
the unified expression as in case of entropy-type in the following way:
separately, one can study them jointly. Let us write these measures in
the unified expression as in case of entropy-type in the following way:
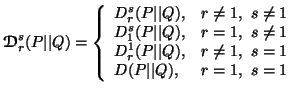 |
(4.1) |
for all ![]() and
and ![]() .
.
The measure ![]() does not appear in the unified expression because it is already contained
in
does not appear in the unified expression because it is already contained
in![]() as a particular case when
as a particular case when ![]() .
.
We call the measure ![]() ,
the unified
,
the unified ![]() relative
information.
relative
information.
Note 4.1. The definition of ![]() and
and ![]() given initially by Sharma and Mittal (1977) [91]
involve
given initially by Sharma and Mittal (1977) [91]
involve ![]() .
But, here in our study we have relaxed this condition. The constant initially
considered was
.
But, here in our study we have relaxed this condition. The constant initially
considered was![]() .
Also, we have changed the multiplicative constant and took it as
.
Also, we have changed the multiplicative constant and took it as ![]() in order to simplify our study on generalized divergence measures. Some
study on the measures
in order to simplify our study on generalized divergence measures. Some
study on the measures ![]() ,
, ![]() and
and![]() can be seen in Mathai and Rathie (1975). [71]
can be seen in Mathai and Rathie (1975). [71]
In particular, we have
|
|
(4.2) |
for all ![]() ,
, ![]() ,
where
,
where ![]() is the unified
is the unified ![]() entropy
given in Chapter 3, expression (3.8).
entropy
given in Chapter 3, expression (3.8).
As in case of generalized entropies, here also we can write
|
|
(4.3) |
where ![]() (reals) is given by
(reals) is given by
![$\displaystyle {\ensuremath{\boldsymbol{\mathscr{N}}}}_s(x)=\left\{\begin{array}......(1-2^{1-s})^{-1}\big[2^{(s-1)x}-1\big], & s\neq 1\\ x, & s=1\end{array}\right.$](img959.gif) |
(4.4) |
Proposition 4.1. The measure ![]() given by (4.4) has the following properties.
given by (4.4) has the following properties.