Go to:Table of Contents

Properties of M-Dimensional Unified (r,s)-Divergence Measures

In this subsection we shall give some properties of $M-$dimensional unified $(r,s)-$measures$^\alpha{\ensuremath{\boldsymbol{\mathscr{I}}}}^s_r(P_1,...,P_M)$, $^\alpha{\ensuremath{\boldsymbol{\mathscr{J}}}}^s_r(P_1,...,P_M)$ and$^\alpha{\ensuremath{\boldsymbol{\mathscr{T}}}}^s_r(P_1,...,P_M)$ ($\alpha=1,2$ and 3).

Property 5.1. The measures $^\alpha{\ensuremath{\boldsymbol{\mathscr{I}}}}^s_r(P_1,...,P_M)$ ($\alpha =1$ and 2), $^\alpha{\ensuremath{\boldsymbol{\mathscr{J}}}}^s_r(P_1,...,P_M)$ ($\alpha=1,2$ and 3) and $^\alpha{\ensuremath{\boldsymbol{\mathscr{T}}}}^s_r(P_1,...,P_M)$ ($\alpha=1,2$ and 3) are nonnegative for all $r>0$ and any $s \in (-\infty,\infty)$ are zero iff$P_1=P_2=...=P_M$. The nonnegativity of $^3{\ensuremath{\boldsymbol{\mathscr{I}}}}^s_r(P_1,...,P_M)$ follows under the conditions when either$s\geq 2-{1\over r}$ or $s\geq r$ with $r>0$.

Property 5.2. For all $r\ \in\ (0,\infty),\ s\\in\ (-\infty,\infty)$, we have 

$$^1{\ensuremath{\boldsymbol{\mathscr{I}}}}^s_r(P_1,...,P_M)\left\{......\boldsymbol{\mathscr{I}}}}^s_r(P_1,...,P_M), & \ s \geq r\end{array}\right.$$ $$\sum_{{j,k=1}_{j\neq k}}^M{\lambda_j\lambda_k}\^1{\ensuremath{\......\boldsymbol{\mathscr{J}}}}^s_r(P_1,...,P_M), & \ s \geq r\end{array}\right.$$ and  $$^1{\ensuremath{\boldsymbol{\mathscr{T}}}}^s_r(P_1,...,P_M)\left\{......\boldsymbol{\mathscr{T}}}}^s_r(P_1,...,P_M), & \ s \geq r\end{array}\right.$$

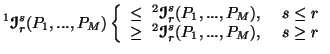
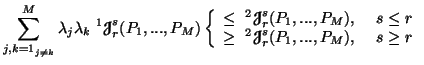
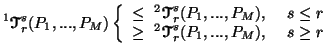
Property 5.3. For all $r\ \in\ (0,\infty),\ s\\in\ (-\infty,\infty)$, we have 

$$^3{\ensuremath{\boldsymbol{\mathscr{J}}}}^s_r(P_1,...,P_M)\geq \^1{\ensuremath{\boldsymbol{\mathscr{I}}}}^s_r(P_1,...,P_M),$$ and  $$^3{\ensuremath{\boldsymbol{\mathscr{J}}}}^s_r(P_1,...,P_M)\geq \ ^3{\ensuremath{\boldsymbol{\mathscr{T}}}}^s_r(P_1,...,P_M).$$

Property 5.4. For all $r\ \in\ (0,\infty),\ s\\in\ (-\infty,\infty)$, $\alpha =1$ and 2, we have 

$$^\alpha{\ensuremath{\boldsymbol{\mathscr{J}}}}^s_r(P\vert\vert Q)...... ^\alpha{\ensuremath{\boldsymbol{\mathscr{I}}}}^s_r(P\vert\vert Q),\ s \geq r>0$$

$$^\alpha{\ensuremath{\boldsymbol{\mathscr{J}}}}^s_r(P\vert\vert Q)\geq 2\ ^\alpha{\ensuremath{\boldsymbol{\mathscr{I}}}}^s_r(P\vert\vert Q).$$

Property 5.5. The measures $^\alpha{\ensuremath{\boldsymbol{\mathscr{I}}}}^s_r(P_1,...,P_M)$ ($\alpha$=1 and 2), $^\alpha{\ensuremath{\boldsymbol{\mathscr{J}}}}^s_r(P_1,...,P_M)$ ($\alpha$=1,2 and 3) and $^\alpha{\ensuremath{\boldsymbol{\mathscr{T}}}}^s_r(P_1,...,P_M)$ ($\alpha$=1,2 and 3) are increasing functions of $r$ ($s$ fixed) and of $s$ ($r$ fixed). In particular, when $r=s$, the result still holds.

Property 5.6. For $r$$\in\$(0,$\infty$), $s$$\in\$($-\infty,\infty$), $\alpha$=1 and 2, the measures$^\alpha{\ensuremath{\boldsymbol{\mathscr{I}}}}(P_1,...,P_M)$, $^\alpha{\ensuremath{\boldsymbol{\mathscr{J}}}}(P_1,...,$$P_M)$ and $^\alpha{\ensuremath{\boldsymbol{\mathscr{T}}}}(P_1,...,P_M)$ are Schur-convex functions of $(P_1,...,P_M)\ \in\ \Delta^M_n$ i.e., $(P_1,...,P_M)\prec (Q_1,...,Q_M)$ implies 

$$^\alpha{\ensuremath{\boldsymbol{\mathscr{I}}}}^s_r(P_1,P_2,...,P_M)\leq\ ^\alpha{\ensuremath{\boldsymbol{\mathscr{I}}}}^s_r(Q_1,Q_2,...,Q_M),$$ $$^\alpha{\ensuremath{\boldsymbol{\mathscr{J}}}}^s_r(P_1,P_2,...,P_M)\leq\ ^\alpha{\ensuremath{\boldsymbol{\mathscr{J}}}}^s_r(Q_1,Q_2,...,Q_M)$$ and  $$^\alpha{\ensuremath{\boldsymbol{\mathscr{T}}}}^s_r(P_1,P_2,...,P_M)\leq\ ^\alpha{\ensuremath{\boldsymbol{\mathscr{T}}}}^s_r(Q_1,Q_2,...,Q_M).$$

Property 5.7. (Generalized data processing inequalities). For $r$$\in\$ (0,$\infty$), $s$$\in\$ ($-\infty,\infty$), $\alpha$=1 and 2, we have 

$$^\alpha{\ensuremath{\boldsymbol{\mathscr{I}}}}^s_r(P_1(B),P_2(B),......M(B))\leq\ ^\alpha{\ensuremath{\boldsymbol{\mathscr{I}}}}^s_r(P_1,P_2,...,P_M),$$

$$^\alpha{\ensuremath{\boldsymbol{\mathscr{J}}}}^s_r(P_1(B),P_2(B),......_M(B))\leq\ ^\alpha{\ensuremath{\boldsymbol{\mathscr{J}}}}^s_r(P_1,P_2,...,P_M)$$ and  $$^\alpha{\ensuremath{\boldsymbol{\mathscr{T}}}}^s_r(P_1(B),P_2(B),......M(B))\leq\ ^\alpha{\ensuremath{\boldsymbol{\mathscr{T}}}}^s_r(P_1,P_2,...,P_M),$$ where $$P_j(B)=\Big(\sum_{i=1}^n{p_{ji}b_{1i}},\sum_{i=1}^n{p_{ji}b_{2i}},...,\sum_{i=1}^n{p_{ji}b_{mi}}\Big)\\in\ _r\Delta_n,\ \forall j=1,2,...,M$$