3. Topological and étale inverse semigroupoids
We will now consider semigroupoid endowed with topologies which are compatible with their algebraic structures. In general, a (partial) algebraic structure $\mathscr{A}$ consists of families $\mathscr{A}_0$ of sets and $\mathscr{A}_1$ of partial functions between sets in $\mathscr{A}_0$, and $\mathscr{A}$ is said to be topological if all sets in $\mathscr{A}_0$ are endowed with topologies, and all functions in $\mathscr{A}_1$ are continuous. The homomorphisms between such structure are also assumed to be continuous. This applies, in particular, to graphs and (Exel/graphed/inverse) semigroupoids. We write the following definitions in full for the sake of completeness.A topological graph is a graph $(G^{(0)},G,\so,\ra)$, such that $G^{(0)}$ and $G$ are endowed with topologies making $\so$ and $\ra$ continuous.
A topological (Exel) semigroupoid is a semigroupoid $\Lambda$ endowed with some topology which makes the product continuous (where $\Lambda^{[2]}$ is endowed with the product topology of $\Lambda\times\Lambda$).
A topological graphed semigroupoid is a graphed semigroupoid which is both a topological graph and a topological semigroupoid.
A topological inverse semigroupoid is an inverse semigroupoid $\mathcal{S}$ which is a topological graphed semigroupoid, and such that the inverse map $(\ )^*\colon\mathcal{S}\to\mathcal{S}$, $s\mapsto s^*$, is continuous.
Note that since $(\ )^*\circ (\ )^*=\id_{\mathcal{S}}$, then $(\ )^*$ being continuous implies that it is a homeomorphism.
A topological inverse semigroupoid $\mathcal{S}$ is étale if the source map $\so\colon\mathcal{S}\to\mathcal{S}^{(0)}$ is a local homeomorphism. (Equivalently, the range map $\ra=\so\circ(\ )^*$ is a local homeomorphism.)
As in [MR2304314], recall that a topological groupoid $\mathcal{G}$ is étale if the source map $\so\colon a\mapsto a^{-1}a$ is a local homeomorphism from $\mathcal{G}$ to $\mathcal{G}^{(0)}=\so(\mathcal{G})$, where $\mathcal{G}^{(0)}$ is endowed with the subspace topology of $\mathcal{G}$. It is not immediately clear that this coincides with the notion in Definition 3.3 since for étale semigroupoids we do not assume $\mathcal{S}^{(0)}$ to even be a subset of $\mathcal{S}$.
Corollary 3.6 below deals with this: Suppose $\mathcal{G}$ is a topological groupoid, étale in the sense of 3.3. Let $\tau$ be the topology of $\mathcal{G}$, $\tau|_{\mathcal{G}^{(0)}}$ ist restriction to $\mathcal{G}^{(0)}$, and $\eta$ the topology of $\mathcal{G}^{(0)}$. By 3.6(a), $E(\mathcal{G})=\mathcal{G}^{(0)}$ is open in $\mathcal{G}$. The source map on $\mathcal{G}^{(0)}$ is simply the identity map $(\mathcal{G}^{(0)},\tau|_{\mathcal{G}^{(0)}})\to(\mathcal{G}^{(0)},\eta)$, and it is a bijective (local) homeomorphism, hence $\tau|_{\mathcal{G}^{(0)}}=\eta$.
Homomorphisms of topological semigroupoids are always assumed to be continuous. A homomorphism $\phi=(\phi^{(0)},\phi^{(1)})\colon G\to H$ of topological graphs is continuous in the sense that $\phi^{(0)}$ and $\phi^{(1)}$ are continuous. When dealing with topological graphed semigroupoids this is a condition which we need to require, but when dealing with étale inverse semigroupoids, continuity of the “arrow map” actually implies continuity of the “vertex map”. For this we recall a fact from the general theory of étale spaces.
Suppose that $\pi_i\colon X_i^{(1)}\to X_i^{(0)}$ ($i=1,2$) are continuous bundles, and that $f=(f^{(0)},f^{(1)})\colon\pi_1\to\pi_2$ is a bundle homomorphism (possibly discontinuous a priori). Suppose further that $f^{(1)}$ is continuous and that $\pi_1$ is open (and surjective). Then $f^{(0)}$ is continuous. Indeed, since $\pi_1$ is open and surjective then $X_1^{(0)}$ is endowed with the the quotient (final) topology that $\pi_1$ induces. We have the commutative square
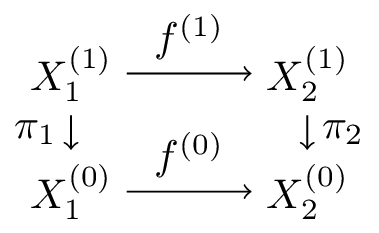
We thus apply the fact above with the bundle induced by the source maps of graphed semigroupoids, and in conjunction with Corollary 2.20 to obtain the result below:
Suppose that $\phi\colon\mathcal{S}\to\mathcal{T}$ is a continuous homomorphism between topological inverse semigroupoids and that $\mathcal{S}$ is étale. Then there exists a unique continuous map $\phi^{(0)}\colon\mathcal{S}^{(0)}\to\mathcal{T}^{(0)}$ for which $(\phi^{(0)},\phi)$ is a graphed semigroupoid homomorphism.
A bisection of a graphed semigroupoid $\mathcal{S}$ is a subset $U\subseteq\mathcal{S}$ such that the source and range maps are injective on $U$. We denote by $\mathbf{B}(\mathcal{S})$ the set of all open bisections of an étale inverse semigroupoid $\mathcal{S}$. If $U\in\mathbf{B}(\mathcal{S})$, then the source and range maps restrict to homeomorphisms from $U$ onto open subsets of $\mathcal{S}^{(0)}$. Moreover, $\mathbf{B}(\mathcal{S})$ is a basis for the topology of $\mathcal{S}$.
If $\mathcal{S}$ is an étale inverse semigroupoid then the product map $\mu\colon\mathcal{S}^{(2)}\to\mathcal{S}$ is open.
We follow the proof of [cordeirothesis]: Let $\pi\colon \mathcal{S}^{(2)}\to\mathcal{S}$ be the projection $\pi(a,b)=a$. We prove that $\pi$ is open. If $A,B\in\mathbf{B}(\mathcal{S})$, then $\pi((A\times B)\cap \mathcal{S}^{(2)})=A\cap \so^{-1}(\ra(B))$. Since $\ra(B)$ is open in $\mathcal{S}^{(0)}$ and $\so$ is continuous, then $\pi((A\times B)\cap \mathcal{S}^{(2)})$ is open in $\mathcal{S}$. This proves that $\pi$ is open, as $\mathbf{B}(\mathcal{S})$ is a basis for $\mathcal{S}$. Moreover, $\pi$ is injective on $(A\times B)\cap\mathcal{S}^{(2)}$, because the range map is injective on $B$. Therefore, $\pi$ is a locally injective open continuous map, that is, a local homeomorphism.
Since $\ra\circ\pi=\ra\circ\mu$ on $\mathcal{S}^{(2)}$ and both $\ra$ and $\pi$ are local homeomorphisms, then $\mu$ is a local homeomorphism as well.
We thus make $\mathbf{B}(\mathcal{S})$ into a semigroup with the usual product of sets: for all $A,B\in\mathbf{B}(\mathcal{S})$,
In Section 5, we will prove that an inverse semigroupoid $\mathcal{S}$ may be recovered from $\mathbf{B}(\mathcal{S})$ and set inclusion $\subseteq$.
We finish this section by mentioning a few simple, but nevertheless important, consequences of Proposition 3.5.
Let $\mathcal{S}$ be an étale inverse semigroupoid. Then
-
$E(\mathcal{S})$ is open in $\mathcal{S}$;
-
For all open $A\subseteq \mathcal{S}$, the upper and lower closures
\begin{equation*} A^{\uparrow,\leq}=\left\{b\in\mathcal{S}:b\geq a\text{ for some }a\in A\right\}\quad\text{and}\quad A^{\downarrow,\leq}=\left\{b\in\mathcal{S}:b\leq a\text{ for some }a\in A\right\} \end{equation*}are open in $\mathcal{S}$.
-
Simply note that $E(\mathcal{S})=\bigcup\left\{A^*A:A\in \mathbf{B}(\mathcal{S})\right\}$.
-
The lower set $A^{\downarrow,\leq}=AE(\mathcal{S})$ is the product of open sets, hence open.
Suppose $b\geq a$ for some $a\in A$, i.e., $ba^*a=a$. Since the semigroupoid operations are continuous, there exist neighbourhoods $U$ of $b$ and $V$ of $a$ such that $UV^*V\subseteq A$. Let $B=U\cap\so^{-1}(\so(A\cap V))$. Then $B$ is an open neighbourhood of $b$.
Given $c\in B$, we have $\so(c)=\so(a)$ for some $a\in A\cap V$, so $ca^*a$ is defined, belongs to $UV^*V\subseteq A$ and $ca^*a\leq c$, so $c\in A^{\uparrow,\leq}$. Therefore $B$ is an open neighbourhood of $b$ contained in $A^{\uparrow,\leq}$.
For non-étale semigroupoids, upper (and lower) closures of open sets are not necessarily open.
Let $X=[0,1]$ with its usual topology and $L_2=\left\{0,1\right\}$ the lattice with $0\smallerthan 1$. The inverse semigroupoid $L_2\times X$ is étale. Consider the subsemigroupoid $\Lambda=(L_2\times X)\setminus\left\{(0,1/n):n\in\mathbb{N}_{\geq 1}\right\}$. Then $\Lambda$ is a non-étale topological inverse semigroupoid.
Let $U$ be any neighbourhood of $0$ in $X$. Then $\tilde{U}\defeq(\left\{0\right\}\times U)\cap\Lambda$ is a neighbourhood of $(0,0)$ in $\Lambda$, but the upper closure $\tilde{U}^{\uparrow,\leq}=(L_2\times U)\cap\Lambda$ contains the non-interior point $(1,0)$.
It follows from Corollary 3.6 that if $\mathcal{S}$ is an étale inverse semigroupoid, then $E(\mathcal{S})$ is also an étale inverse semigroupoid with the subspace topology. In fact a weaker version of the converse holds: If $\mathcal{S}$ is a topological inverse semigroupoid and $E(\mathcal{S})$ is open and étale, then $\mathcal{S}$ has a basis of open bisections. However this only implies that the source map is locally injective, but not necessarily open, and so $\mathcal{S}$ may be non-étale. In any case, the following generalization of [MR2304314] holds, with simple adaptations on the proof.
Let $\mathcal{S}$ be a topological inverse semigroupoid. Then the following are equivalent:
-
$\mathcal{S}$ is étale;
-
$E(\mathcal{S})$ is open and étale, and the product of open sets is open.
-
$E(\mathcal{S})$ is open and étale, and $\mathcal{S}A$ is open for each open $A\subseteq\mathcal{S}$.
-
$E(\mathcal{S})$ is open and étale, and $A^*A$ is open for each open $A\subseteq\mathcal{S}$.
-
$E(\mathcal{S})$ is open and étale, and the source map is open;
3.1. Continuous $\land$-preactions and semidirect products
Tipically, in the topological setting we consider actions which preserve the topological structure. Let us consider the “action map” associated to a $\land$-preaction $(\pi,\theta)\colon\mathcal{S}\curvearrowright\Lambda$, which we also denote by $\theta$:
A $\land$-preaction $(\pi,\theta)\colon\mathcal{S}\curvearrowright\Lambda$ of a topological inverse semigroupoid $\mathcal{S}$ on a topological semigroupoid $\Lambda$ is continuous if $\pi$ and the “action map” $\theta\colon\mathcal{S}\ltimes\Lambda\to\Lambda$ are continuous. If the action map $\theta$ is open then we call $(\pi,\theta)$ an open $\land$-preaction.
Evidently, the semidirect product $\mathcal{S}\ltimes\Lambda$ associated to a continuous $\land$-preaction $(\pi,\theta)\colon\mathcal{S}\curvearrowright\Lambda$ is a topological semigroupoid (as long as the product (2.49) is associative).
Suppose that $(\pi,\theta)\colon\mathcal{S}\curvearrowright\Lambda$ is a continuous open $\land$-preaction. Given $a\in\mathcal{S}$, let $A$ be any open bisection containing $a$. Then
If $\Lambda$ is a topological graphed or inverse semigroupoid then $\mathcal{S}\ltimes\Lambda$ will also be a topological graphed or inverse semigroupoid, where the vertex set $(\mathcal{S}\ltimes\Lambda)^{(0)}=\Lambda^{(0)}$ is endowed with its original topology.
The following proposition simplifies the verification of when an action is open.
Let $(\pi,\theta)\colon\mathcal{S}\curvearrowright\Lambda$ be a continuous $\land$-preaction of topological semigroupoids. Then $(\pi,\theta)$ is open if and only if the map
Let $A\subseteq\mathcal{S}$ and $U\subseteq\Lambda$ be open. Assuming that $(\pi,\theta)$ is open, we have
Conversely, assume that $p$ is open. The map
For example, if $(\pi,\theta)\colon\mathcal{S}\curvearrowright\Lambda$ is a continuous $\land$-preaction and $\mathcal{S}\ltimes\Lambda$ is open in $\mathcal{S}\times\Lambda$, then $(\pi,\theta)$ is also open. This is an assumption made, for example, in [MR2045419].)
Finally, if $\mathcal{S}$ and $\mathcal{T}$ are étale inverse semigroupoids and $(\pi,\theta)\colon\mathcal{S}\curvearrowright\mathcal{T}$ is an open continuous $\land$-preaction, then $\mathcal{S}\ltimes\mathcal{T}$ is also an étale inverse semigroupoid. Indeed, if $A\subseteq\mathcal{S}$ is an open bisection and $U\subseteq\mathcal{T}$ is open, then the map $p$ is injective on $A\ast U$. Indeed, if $p(a,x)=p(b,y)$, where $(a,x),(b,y)\in A\ast U$, then $x=y$ (by definition of $p$) and $\so(a)=\pi(x)=\pi(y)=\so(b)$, thus $a=b$ as $A$ is a bisection. Therefore $p$ is locally injective, continuous and open, i.e., a local homeomorphism. The range map $\ra_{\mathcal{S}\ltimes\mathcal{T}}$ of $\mathcal{S}\ltimes\mathcal{T}$ is the composition of the range map $\ra_{\mathcal{T}}$ of $\mathcal{T}$ and the action map $\theta$, which are both local homeomorphisms. Therefore $\mathcal{S}\ltimes\mathcal{T}$ is étale.
We will now describe an analogue, in the setting of semigroupoids, of the canonical actions of a semigroup on its idempotent semilattice (the Munn representation), and of
Let $\mathcal{S}$ be an inverse semigroupoid. We denote by $\operatorname{F}(\mathcal{S})$ the set of elements $b\in\mathcal{S}$ such that
-
$\so(b)=\ra(b)$;
-
For all $e\leq b^*b$, we have $beb^*=e$;
The verification that $\operatorname{F}(\mathcal{S})$ is an inverse sub-semigroupoid of $\mathcal{S}$ is straightforwards, using properties of the canonical order of $\mathcal{S}$. Note that $E(\mathcal{S})\subseteq\operatorname{F}(\mathcal{S})$. If $b\in\operatorname{F}(\mathcal{S})$, then $b^*b=bb^*$, and for all $e\in E(\mathcal{S})\cap\mathcal{S}^b$, $beb^*=eb^*b$ If $a\in\mathcal{S}$, $b\in\operatorname{F}(\mathcal{S})$ and $ab$ is defined, then $aba^*\in\operatorname{F}(\mathcal{S})$ as well.
Then $\mathcal{S}$ carries a natural action by conjugation) on $\operatorname{F}(\mathcal{S})$ as follows. Consider the bundle $\pi\defeq\so|_{\operatorname{F}(\mathcal{S})}=\ra|_{\operatorname{F}(\mathcal{S})}\colon\operatorname{F}(\mathcal{S})\to\mathcal{S}^{(0)}$. Given $a\in\mathcal{S}$, let $\dom(\mu_a)\defeq\left\{b\in\operatorname{F}(\mathcal{S}):bb^*\leq a^*a\right\}$, which is an ideal of $\operatorname{F}(\mathcal{S})$, and contained in $\pi^{-1}(\so(a))$. The map $\mu_a\colon\dom(\mu_a)\to\ran(\mu_{a^*})$, $\mu_a(b)=aba^*$, is an isomorphism, and the pair $(\pi,\tau)$ is a global action of $\mathcal{S}$ on $\operatorname{F}(\mathcal{S})$.
If $\mathcal{S}$ is an étale inverse semigroupoid, then $\operatorname{int}(\operatorname{F}(\mathcal{S})$ is invariant, in the sense that $\mu(\mathcal{S}\ast\operatorname{int}(\operatorname{F}(\mathcal{S})))\subseteq \operatorname{int}(\operatorname{F}(\mathcal{S}))$. Indeed, if $A$ and $U$ are open bisections of $\mathcal{S}$ and $U\subseteq\operatorname{F}(\mathcal{S})$, then $\mu(A\ast U)=AUA^*$ is the set of products $aua^*$ (whenever defined), where $a\in A$ and $u\in U$. Since the product of open sets is open and $\mathcal{S}\ast\operatorname{int}(\operatorname{F}(\mathcal{S}))$ is the union of all such sets $A\ast U$, then $\operatorname{int}(\operatorname{F}(\mathcal{S}))$ is invariant.
Thus we may restrict the action $(\pi,\mu)$ to $\operatorname{int}(\operatorname{F}(\mathcal{S}))$. In fact, the argument in the paragraph above proves that the restriction of $(\pi,\mu|_U)$ to any invariant open subset $U$ of $\operatorname{F}(\mathcal{S})$ is an open action. Since $E(\mathcal{S})$ is also open and invariant, then the semigroupoids $\mathcal{S}\ltimes\operatorname{int}(\operatorname{F}(\mathcal{S})$ and $\mathcal{S}\ltimes E(\mathcal{S})$ are étale as well.
In classical cases, the action $\mu$ is well-known.
-
If $\mathcal{S}$ is an étale groupoid, then $\operatorname{F}(\mathcal{S})$ is the isotropy subgroupoid of $\mathcal{S}$. The restriction of $(\pi,\mu)$ to $E(\mathcal{S})=\mathcal{S}^{(0)}$ is the canonical action of $\mathcal{S}$ on its unit space: $\mu_g$ is defined only as $\mu_g(\so(g))=\ra(g)$, for each $g\in\mathcal{S}$.
-
if $\mathcal{S}$ is a discrete inverse semigroup then the restriction of $(\pi,\mu)$ to $E(\mathcal{S})$ is the Munn representation of $\mathcal{S}$.
We finish this section by proving that continuous $\land$-preactions of discrete inverse semigroups, and continuous non-degenerate global actions of étale groupoids, are always open. Of course, it is enough to consider only actions on topological spaces (unit groupoids).
Just as in Example 2.43, a topological partial (or global) action $\theta$ of an inverse semigroup $S$ on a topological space $X$ is precisely a continuous partial action of $S$ on $X$, as topological semigroupoids (as in [arxiv1804.00396]), where we regard $S$ as a discrete space.
In fact, more generally, if $\theta$ is a continuous $\land$-preaction of $S$ on $X$, then $\dom(\theta_a)$ is open in $X$ for all $a\in S$ (by a previous remark). Thus $S\ltimes X=\bigcup_{a\in S}\left\{a\right\}\times\dom(\theta_a)$ is open in $S\times X$ (since we regard $S$ as a discrete space). From the comment after Proposition 3.10, it follows that $\theta$ is an open $\land$-preaction, and in particular $S\ltimes X$ is an étale inverse semigroupoid.
As in Example 2.44, a continuous, non-degenerate global action $(\pi,\theta)\colon\mathcal{G}\curvearrowright X$ of a topological groupoid $\mathcal{G}$ on a topological space $X$ is the same notion as used in [MR2969047]. Suppose moreover that $\mathcal{G}$ is étale, and let us prove that $(\pi,\theta)$ is open. The map $p$ of Proposition 3.10 is precisely the source map $\so\colon\mathcal{G}\ltimes X\to X$, $\so(g,x)=x$, so we need only to prove that it is open. Let $A\subseteq\mathcal{G}$ and $U\subseteq X$ be open. From Example 2.44, we know that $\pi^{-1}(\so(a))=\dom(\theta_a)$ for all $a\in\mathcal{G}$, so it follows that
We now refine Proposition 2.48 to the topological setting. Recall that any $\land$-preaction $(\pi,\theta)$ may be extended, in a minimal manner, to a partial action $(\pi,\overline{\theta})$.
If $\mathcal{S}$ is an étale semigroupoid, $\Lambda$ is a topological semigroupoid and $(\pi,\theta)$ is a continuous (resp. open) $\land$-preaction, then the minimal partial action $(\pi,\overline{\theta})$ of Proposition 2.48 is also continuous (resp. open).
To avoid confusion, we denote $\mathcal{S}\ltimes\Lambda$ and $\mathcal{S}\overline{\ltimes}\Lambda$ the (underlying sets of the) semidirect products associated to $\theta$ and $\overline{\theta}$, respectively, and if $A\subseteq\mathcal{S}$, $U\subseteq\Lambda$, by $A\ast U\defeq(A\times U)\cap(\mathcal{S}\ltimes\Lambda)$ and $A\overline{\ast}U\defeq(A\times U)\cap(\mathcal{S}\overline{\ltimes}\Lambda)$. The action maps are still denoted $\theta$ and $\overline{\theta}$.
If $U\subseteq\Lambda$ is open, then $\overline{\theta}^{-1}(U)$ is the union of the sets of the form $(A^{\uparrow,\leq})\overline{\ast} V$, where $A\subseteq \mathcal{S}$ and $V\subseteq\Lambda$ are open, and $A\ast V\subseteq\theta^{-1}(U)$. Thus, if $(\pi,\theta)$ is continuous then $(\pi,\overline{\theta})$ is also continuous.
Similarly, if $A\subseteq\mathcal{S}$ and $V\subseteq\Lambda$ are open, then $\overline{\theta}(A\overline{\ast} V)=\theta(A^{\downarrow,\leq}\ast V)$, so $(\pi,\theta)$ being open implies that $(\pi,\overline{\theta})$ is also open.